What makes two or more musical notes sound good (or bad) when sounded together? A music teacher can tell you how notes can be combined to sound good- that is what music theory is all about. It is harder to talk about why we perceive those combinations to be satisfying, but that discussion can lead to new and innovative methods for composition and performance.
Background:
This paper was first published in 1986, in the journal 1/1. I have revised it several times, added more graphics, and have written a couple of interactive programs to let you hear the intervals and chords discussed here. I’ve added some introductory material as well–most musicians have not had the time to study musical acoustics in any depth, and the same can be said for many acousticians and the study of music. For further study, there is a considerable library of good reference works on musical acoustics, especially “Horns, Strings, and Harmony” and “Fundamentals of Musical Acoustics“, both by Benade.
For more advanced reading, see the recent book “Tuning, Timbre, Spectrum, Scale” by Sethares (Springer-Verlag), which goes more deeply into the subject, and focuses on the harmonic consequences of non-harmonic partials in musical tones.
Please feel free to add comments and questions here, or mail me at norm-at-sohl-dot-com.
Why study consonance and dissonance?
Composers have always tried to expand their palette of compositional resources. This desire to continually re-invent music theory has shaped western music, and is one of the features that distinguishes our musical tradition. Composers in the 21th century are in a difficult position – the compositional rules of the past have played out, and new theories, techniques and technologies have yet to be proven completely valid (or emotionally satisfying) as compositional resources.
We are in the difficult position of developing new theories while trying to compose music based on those theories. In a way our time as similar to that of early composers such as Leonin and Perotin who formalized an entirely new style of music based on the medieval improvisational art of descant. They pioneered the basic elements of harmony and counterpoint which still serve as the foundation of common-practice composition today. Starting from a strong philosophical tradition, these composers linked their theories to the absolutes of their time such as theology and mathematics. That foundation proved strong – it is only in the last century or so that it has started to play out, and with it the philosophical basis for much of our music theory.
My composition teacher was trained in the 1950s, and taught the conventional wisdom of the time that consonance was a variable cultural value and was in no way absolute. I studied in the 1980s at a time when new research was showing that consonance and dissonance do exist as physical phenomena, and are to some degree constant across cultural boundaries. It’s that belief that I carry forward here.
Most anyone, child or adult recognizes the octave, the fifth, and basic elements of rhythm without any particular musical training, just as they recognize simple visual patterns and colors. Not only do these musical patterns remain constant, but music around the world is based on them.
One challenge to the modern composer is that these patterns are too well understood. It’s hard to find new musical structures which are based on these elements yet go somewhere new and interesting. In this paper I’ve tried to go back to basics to study the physical elements of consonance, dissonance and rhythm, hoping that this will suggest some new and interesting compositional directions.
I suspect that new compositional rules for harmony (and to a great extent melody and rhythm) can be deduced from a good basic understanding of the acoustic and perceptual principles that underlie consonance and dissonance. This paper makes no attempt to provide that basis but takes it as fact, and tries to make some new observations based on that understanding.
Consonance-two musical tones sounding together
What is consonance?
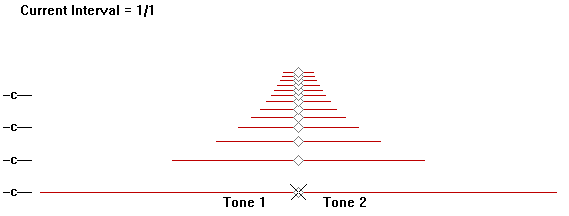
Consonance means “to sound together”, and it occurs in music when two musical tones are played together where the pitch of the tones have certain mathematical relationships. It was well known in the middle ages that simple consonant intervals could be played between two strings by letting one string sound its full length, and stopping the other string at 1/2, 2/3, and 3/4 of it’s length . These intervals are usually called “just” and they are usually referred to as ratios:
- 1/1 has both strings open and sounding the same note to produce a unison
- 2/1 produces the interval of the octave. One string is 1/2 the length of the other
- 3/2 produces the interval of the 5th
- 4/3 produces the 4th
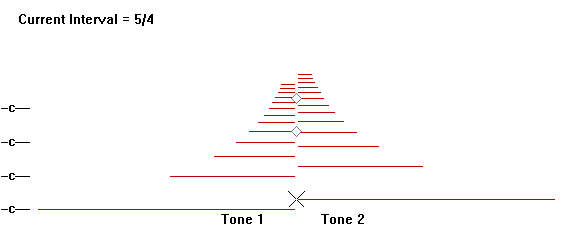
- 5/4 produces the major 3rd
- and so on…
It took until the 1800’s for a reasonable scientific explanation of why this should be so. The German scientist Hermann Helmholtz published “On the Sensation of Tone” where he suggested that these consonant intervals were caused by the alignment of the harmonics of the 2 musical tones being produced by the strings.
Even though parts of Helmholtz’s theory have been disproved, his general theory of harmonic interaction remains a useful and empirically sensible model for practical musicians and a common starting point for current research in musical perception. It is the starting point for the discussion in this paper.
So, if consonance is caused by the alignment of the partials of musical tones, what are these partials, and what makes up a musical tone?
What is a “musical” tone?
There are an amazing variety of musical tones. A violin sounds very different from a tuba, and both sound very little like a flute. These musical tones differ because they are made up of different combinations of frequencies (partials) and the mix of those frequencies make up the recipe that in part gives the instrument its unique sound.
The recipe of a musical tone differs from random noise in that its partials for the most part fall into what a mathematician would call a harmonic series. Not surprisingly the harmonic series provides a strong foundation for the ratios of the just intervals discussed above. “Horns, Strings, and Harmony” by Benade is a textbook on musical acoustics that goes into great depth on the subject.
Every musical tone is different, made up of harmonic partials of different strengths, and many (like bells) include partials that don’t fall on the harmonic series. In order to keep things simple, I am going to consider an “idealized” musical tone, one where all the partials are harmonic, their number is limited, and their intensity drops off in a regular way.
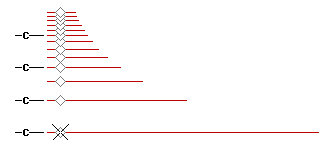
Spectrum (recipe) of an idealized musical tone.
In this chart, the vertical axis of the graph is pitch (marked in black for octaves of c) and the red bars are the partials that make up the tone. The horizontal axis represents the strength of the harmonic partial.
Consonance between partials
How is consonance related to the harmonic partials of a musical tone? Let’s return to strings for a moment. Imagine that we have two strings tuned to the same pitch. It should be obvious that all the significant partials of these two musical tones all align, so the notes produced by the two strings sound in tune. This produces the musical interval of 1/1, also called unison. Here is a diagram of the partials of the two notes compared back to back.
Two tones at the same pitch.
If we use a slide to shorten one of the strings the pitch of the shortened string will go higher, and the strings begin to go out of tune because the partials no longer align. This produces unpleasant “interference tones” or beats, which increase in speed as the two pitches move apart. Anyone who has tuned a stringed musical instrument should be familiar with the sound of these beats.
If things just continued to get worse as the distance between the tones increased, there would be no such thing as music. Fortunately, that is not the case. As the two musical tones get further apart, different harmonic partials come back into alignment and sound together. That is what the word consonance means – to sound together.
In the next example, the 4th partial of one tone aligns with the 5th partial of the other tone creating the interval of a 5/4, the major third. I’ve added diamonds where the partials precisely align.
Moving on, then the 3rd aligns with the 4th for the interval of the fourth.
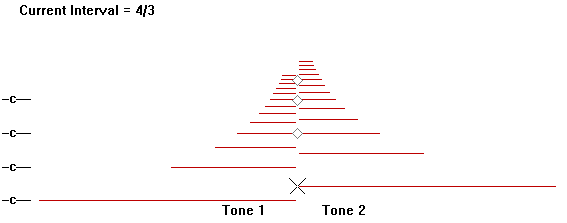
The 2nd aligns with the 3rd, giving the interval of the perfect 5th.
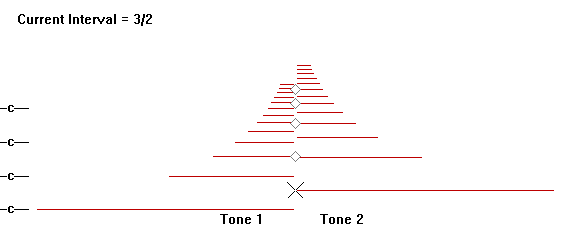
and finally the 1st aligns with the 2nd to give the interval of the octave.
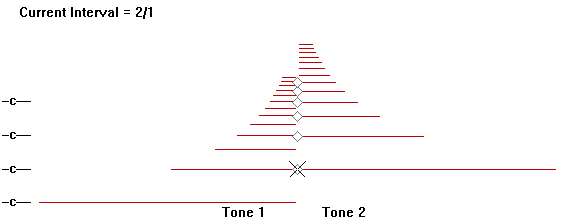
There can be many more partials in a musical tone and more alignments exist than is shown here.
Scales: a collection of consonances
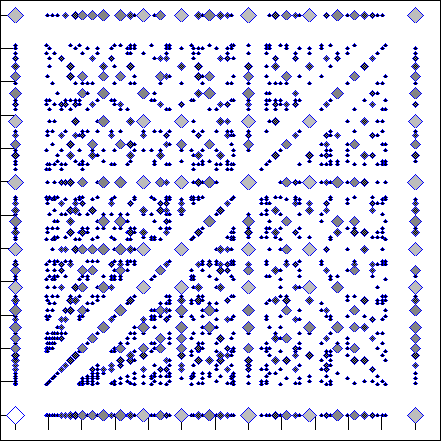
A scale is simply a collection of intervals. The 12 tone (chromatic) scale common to most modern western musical instruments provides an approximation of these consonant intervals. It is a compromise scale, needed to make mechanical instruments play in more than one key.
Today we have the ability to completely control electronic and computer musical instruments (and the associated spectrums of musical sound) and we may be nearing a time when these tuning compromises are no longer needed. The graphs that follow use the letter names of the 12 tone equal temperament scale for reference, and you can see that the notes are approximations, and do not always line up with the just intervals generated.
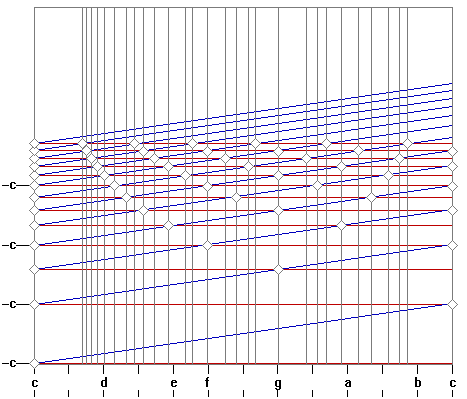
Interactions of two musical tones, one fixed and one moving over the span of an octave.
A musical scale made up of pure consonances is often referred to as a “just” scale. There is nothing particularly modern about this scale-it crops up throughout history and all over the world.
A just scale can be graphed as shown in the figure above. What this represents is a graph of two tones, one moving through the span of an octave against a second fixed tone. As differing harmonics of the two tones match with each other a consonance occurs, and is marked with a diamond. There are quite a few intervals in this scale, since the graph includes quite a few partials. Not all of these intervals line up with the named notes of the 12 tone scale.
In the next diagram, a just scale, with a limit of 6 is shown. It contains all the major consonances used in the 12 tone scale.

Intervals of a just scale with a harmonic limit of 6
(The white diamond on the left represents the note middle C, and the gray diamond on the right represents c at the octave. the black tick marks represent 12 tone equal temperament, and the red marks above the steps of a just scale.)

Intervals of a just scale with a harmonic limit of 7
If we increase the limit to 7, we get a couple of new intervals, including the interval of the flattened minor 7th familiar from blues and jazz. This interval lies outside of the 12 tone scale, and is used only by performers who have instruments that are not locked into a fixed tuning (slide guitar and voice, for example).

Intervals of a just scale with a harmonic limit of 11
Its obvious that calculations that include more harmonics will create scales with many more intervals than the common 12 tone scale can accommodate. Not all of these intervals are really useful, since they are often produced by weak partials and often occur in parts of the scale where they end up masked by dissonances created by other stronger partials (more on that later). Still, there are situations where some of these intervals are very valuable, and can provide considerable new resources for composition.
Chords: consonances between three tones
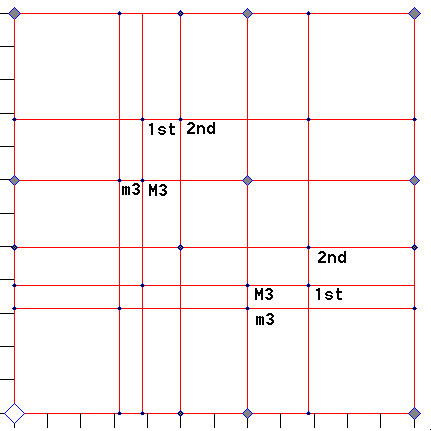
(4) Chords generated by interactions of the 1st. 6 harmonic partials
Each axis of the graph represents the scale generated by one tone moving against a drone, as described above. Every possible combination of these tones–(one drone and two moving tones) within the span of the octave is represented as a point somewhere on the graph. The red lines mark places where the drone and one moving tone form a consonance. The diamonds mark a place where all three tones form consonances with each other.
A grouping of three tones is defined as a chord if all three members of the group share consonant relationships with each other. Obviously, the notes from the two axes share consonance with the bass tone, but in addition, they must be consonant with each other.1
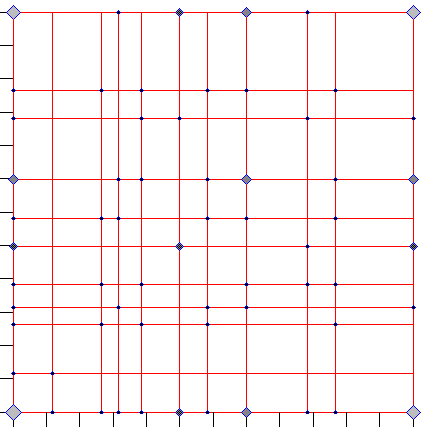
(5) Chords generated by interactions of 7 harmonic partials
If the harmonic limit is expanded to seven (see Figure 5), we have, in addition to the previous chords, an incomplete seventh chord, and several other new chords unique to Just Intonation. Increasing the limit causes more chords to be displayed. Of course there is no reason to stop at 7:
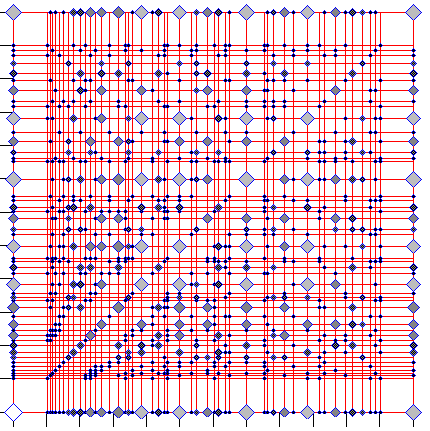
(6) Chords generated by interactions of 17 harmonic partials
Inversions
So far, we have been able to think of all points in this graph as representing the set of all chords sharing a common bass tone (1/1). This graph helps to define consonant chords, and can also be used to compose music involving two moving parts against a drone. It can also be used without a drone, to plot two tones playing in counterpoint, if we accept the just scale melodically as well as harmonically. In this case, the 1/1 is not sounded, except as a melodic interval, but it remains as the tonal center of the piece.
There s not enough space in one article to expand the graph much further, but one important aspect of chord structure can be added, that of inversions of chords with common roots.
Chords below the root
Illustrate
If the graph is expanded to cover two octaves, with 1/1 at the center (now the root, not just the bass tone),second inversions will fall in the lower right as well as the upper left, since these two parts of the graph are mirror images of each other. Inversions of other chords which share a common element with the center tone can also be found.
Chords with 4 tones
Illustrate
The system can be expanded to represent four tones, that is three notes over a drone, by adding a third axis to the graph. This means either constructing a three-dimensional model, or as is more practical, printing a series of graphs representing the planes of the third axis or creating a 3D computer rendering. It should be remembered that all tones must share consonance with the drone or keynote, as well as with each other. This set of graphs can represent all possible consonant combinations of four tones with a particular harmonic limit. Most of these chords involved some form of doubling, but some contain four unique tones.
Implications for chordal composition
As was mentioned above, the 1/1 may act as a drone of even not sound, and simply imply the tonality of the piece. In this case the set of graphs can be used to describe the fully consonant chords of any scale degree in just intonation. As anyone who has tried to play conventional chords against a drone will know, certain common chords clash vividly – obviously these chords will not be marked as consonant in these graphs. This has some serious implications since one of these chords is the major triad to the step 3/2, the V chord, which is typically necessary for cadences. The V-I progression is perhaps the most powerful chord progression in music theory, so it might seem that something is wrong here. The problem lies not with the model, but in the assumption that the V must belong to the same tonality as the I. This cannot be true, since the V contains a tone (call it B natural in the graph of the 3/2) which fills within the region of greatest possible dissonance surrounding the 1/1.
In monophonic writing in the Western tradition, intervals this close to the unison are used to develop a strong drive to that unison. The tension of a strong dissonance near perfect consonance, drives the melody toward union. This explains part of the drive in the V-I progression. The tonality seems to change to allow the V chord in the same way a secondary dominant momentarily shifts the tonality of a piece of conventional harmony.
Chords which span more than one octave
Illustrate
The graph above has been expanded to cover two octaves, and shows all chords with a span of two octaves. One interesting result of a physical definition of consonance is that the octave can no longer be considered invariant. Scale structure is similar across octaves, but more sparse as the distance between the drone tone and the moving tone increases, which creates more potential compositional resources.
Graphs of More than 4 tones
We can add another axis to the four-tone graph to represent either a fifth tone or a tonal center with four tones moving. This is the construct necessary to fully represent four-part writing. The complexity of a four-dimensional graph limits its usefulness as a physical model but in the application of computer-aided composition, those restrictions need not apply. The ease of calculation and generation of chords and scales, and the extendibility of the system to any number of tones makes this method particularly suited for sophisticated computer-aided modeling, analysis, and composition.
Visualizing Counterpoint
Contrary motion, or counterpoint between the two moving tones can be seen as diagonal motion from lower right to upper left, where parallel motion runs contrary to that. Similar motion runs between these extremes.
Melody
This last experiment leaves us on theoretically shaky ground. Because the model of consonance is based on the actual physical interaction of specific sounds, it can only apply directly to the perception of consonance between simultaneous tones, to the vertical aspect of harmony, and not to horizontal structure or melody. We normally assume that the same rules apply to musical intervals when used melodically as when they are used harmonically. It is often possible to think of melodic structure in simpler terms however, since the human ear’s resolution of pitch is much more accurate in terms of harmony than melody.
It is not uncommon to find historical references to strictly harmonic just intonation. For example the debates earlier this century concerning intonation in the playing of string quarters (chap. 9, Intervals, Scales, and Temperaments by Lloyd and Boyle). What concerned these writers was a harmonic Just Intonation, all intervals in tune with each other but within the melodic structure of the twelve-tone scale. A similar idea is advocated by Harold Waage in “The Intelligent Keyboard” (1/1, Vol. 1, No. 4).
The use of the just scale melodically does have some advantages. This creative use of dissonance as well as consonance seems to give music a strong sense of power and movement, and this is one case where it is useful to free melodic movement from the strict scale generated by a drone, or allow modulation of the tonal center. In analysis of conventional music based on this model of tonality, a much greater role must be assigned to these transitory modulations. This idea is not entirely foreign to modern conventional music theory, but its application has never been widespread.
Still, extremely complex and interesting polyphony can be written within a strict definition of tonality. There are other forms of V chords within the strict chart which, although they would be considered incomplete in traditional music, might still perform a dominant function. An example is the V7 lacking the third.
Dissonance
Dissonance is often thought of as the absence of consonance, and in a general way, this is true enough, but there is more to the structure of dissonance which may be of value to a composer.
Rhythm
Rhythm can be thought of as a similar phenomena to consonance as shown in the following experiment: Record a drummer playing a simple series of ¼ notes. As that recording is played back, artificially speed it up. As the beats pass the speed of 30 or so a second, the resulting sound will be perceived as a musical pitch. When the rate reaches 440 a second, the sound of concert A will result. Now repeat that experiment, with the drummer playing a pattern of 2 beats to 3 beats (two on three). When that pattern is played back at a higher rate of speed, the sound of the interval of the 5th is heard! This is not to say that rhythm and consonance are identical phenomena-they are not, but they are similar in some very interesting ways.
Footnotes
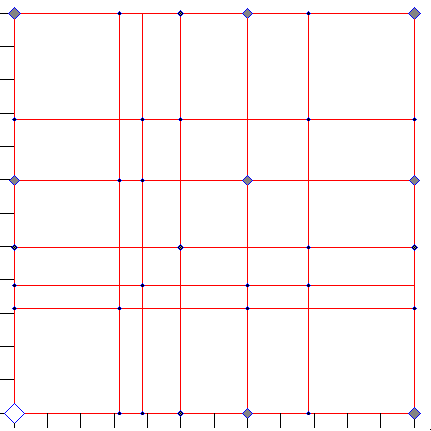
1 It is interesting to note that although this graph is based on the physical structure of sound, there is no problem in generating the minor triad or other chords which have presented problems for previous “physical” theories (for example, see Tone, Levarie and Levy, page 189). In Figure 2, note that the major triad “M3”, the minor triad “m3”, and first and second inversions “1st” and “2nd” all appear.
2 Anyone can perform the following simple experiment to discover consonant intervals: Make a “monochord” by stretching two similar rubber bands over a cardboard box. Place pencils under the bands at each end of one side of the box, so that the bands are held above the box about ¼ inch. Adjust the tension of the bands so that the two “strings” of the monochord produce the same note. Now slide a pencil under only one string, so that the pitch moves up and down
